The chemical percolation devolatilization (CPD) model was developed to model coal devolatilization based on characteristics of the chemical structure of the parent coal. The model is intended to be an engineering model that (a) is suitable for application in large comprehensive models of coal combustion, and (b) accurately models key chemical structural features and reaction mechanisms of coal. The CPD model was originally developed as a stand-alone code, but has also been incorporated into comprehensive coal combustion models such as PCGC-3 and Fluent.

The chemical percolation devolatilization (CPD) model describes the devolatilization behavior of rapidly heated coal based on the chemical structure of the parent coal.[1-3] A summary document is available that provides complete details of the development of the CPD model.[4] The CPD model consists of five principal components:

1. A description of the parent coal structure

2. A bridge reaction mechanism with associated kinetics

3. Percolation lattice statistics to determine the relationship between bridge breaking and detached fragments (these fragments are tar precursors)

4. A vapor-liquid equilibrium mechanism to determine the fraction of liquids that vaporize

5. A cross-linking mechanism for high molecular weight tar precursors to reattach to the char.

Coal Structure

The CPD model is the only devolatilization model which directly uses values of measured chemical structure features. Other current models, such as Niksa's FLASCHAIN[5] and Solomon's FG-DVC[6], use a large degree of empiricism in order to get final answers that look reasonable. The CPD model uses four features of the chemical structure that are directly measured by 13-C NMR spectroscopy:

- The average molecular weight per aromatic cluster (M_cl)

- The average molecular weight per side chain (M_del)

- The average number of attachments (i.e., side chains and bridges) per cluster, referred to as the coordination number (sigma+1)

- The fraction of attachments that are bridges (P0)

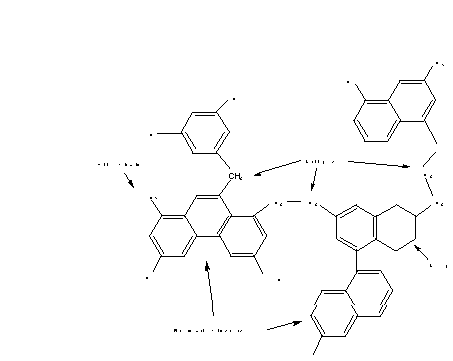
These chemical structure features are illustrated in Figure 1; aromatic clusters are connected by bridges and loops, and side chains serve as gas precursors. In addition, a small amount of char bridges (C0) must be accounted for (a) in lignites to account for early crosslinking, and (b) very high rank coals to account for bi-aryl linkages. At present, measurements of structural features for C0 are not available, and an empirical relationship is used for this one variable. Overall, direct use of 13-C NMR parameters in the CPD model is in contrast to the previous and common practice of adjusting input coefficients to precisely match measured tar and total volatiles yields.

Correlation of NMR Parameters

Since the NMR parameters described above are so useful, and since they are only available for about 30 coals, a correlation was developed to derive the NMR-based coal structure parameters from a combination of the elemental analysis and the proximate analysis (ASTM volatile yield). This work is described by Genneti, Fletcher, andPugmire (Energy and Fuels, 13, 60-68, 1999) and in Genetti's M.S. Thesis (1999).

Figure 1. Representative chemical structures identified in 13-C NMR analyses and used in the description of coal and coal chars in the CPD model.

Bridge Reaction Mechanism

During devolatilization, the bridges between aromatic clusters break; aromatic clusters are thermally stable at typical devolatilization temperatures. The bridge reaction mechanism used in the CPD model is illustrated in Figure 2; an unreacted bridge forms a reactive intermediate, which may either (a) cleave to form two side chains or (b) reconnect to form a stable char bridge with release of part of the bridge as light gas. Reaction rates for this mechanism were obtained by comparison with measured devolatilization rates, and are coal independent (especially at high heating rates). Note that the gas and tar yields are a function of the chemical structure parameters and the reaction scheme, and are therefore not input parameters.

Percolation Lattice Statistics

Percolation lattice statistics are employed to describe the generation of tar precursors of finite size based on the number of cleaved labile bonds in the infinite coal lattice. This is a non-linear relationship, and the percolation lattice statistics provide a closed-form relationship that avoids computationally expensive Monte Carlo technique originally proposed by Solomon.[6] A tree-like structure called a Bethe lattice is used to approximate the coal lattice. The Bethe lattice accounts for the crosslinking present in the parent coal structure, as opposed to the long chain approximation used by Niksa[5]. The Bethe lattice is fully described by the five chemical structure parameters (Mcl, Mdel, sigma+1, P0, and C0).

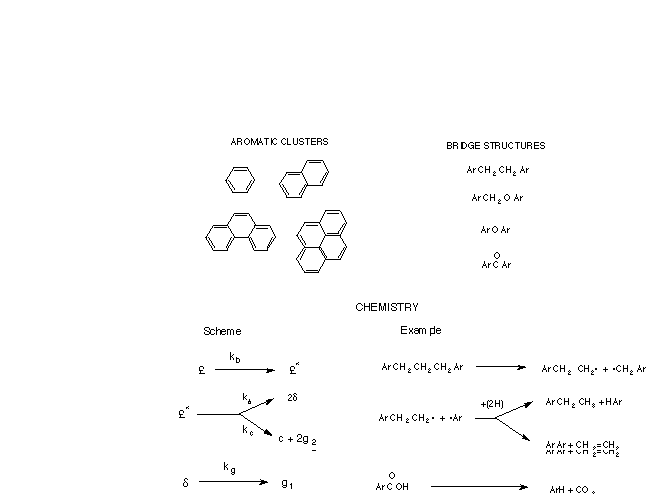
Figure 2. Representative chemical structures corresponding to the chemical reaction scheme in the CPD model.

Vapor-Liquid Equilibrium

A generalized vapor pressure correlation for high molecular weight hydrocarbons, such as coal tar, was developed based on data from coal liquids. The vapor pressure of each oligomer size (monomers, dimers, etc.) are calculated from the temperature and molecular weight at each time step. A flash calculation is performed to determine the fraction of each oligomer size that vaporizes at that time step. The vapor-liquid equilibrium mechanism is principally responsible for the change in tar yield observed as the total pressure is changed in devolatilization experiments. The vapor pressure relationship developed here also agrees well with pure component vapor pressure data of 111 organic compounds thought to exist in coal tar.[3]

Crosslinking mechanism

The crosslinking mechanism permits reattachment of metaplast (i.e., detached finite fragments) to the infinite char matrix. Since details of the crosslinking mechanism are poorly known at present, a simple empirical crosslinking rate is employed that is first order in the amount of metaplast (detached fragments) associated with the char. The coal-independent crosslinking rate was determined by comparison with several sets of data.[3]

The CPD model successfully predicts the effects of pressure on tar and total volatiles yields observed in heated grid experiments for both bituminous coal and for lignite. Predictions of the amount and characteristics of gas and tar from many different coals compare well with available data, as shown in Figure 3, which is unique because the majority of model input coefficients are taken directly from NMR data, rather than used as empirical fitting coefficients. Predicted tar molecular weights are consistent with size-exclusion chromatography (SEC) data and field ionization mass spectrometry (FIMS) data. Predictions of average molecular weights of aromatic clusters as a function of coal type agree with corresponding data from NMR analyses of parent coals.

Figure 3. Predicted and measured tar and total volatiles yields for a wide range of coals. Perfect agreement is illustrated by the 45 degree line. (See [3]).

The direct use of chemical structure data as a function of coal type helps justify the model on a mechanistic rather than an empirical basis. Empirical correlations of input parameters are available, but these correlations tend to smooth out the features of problem coals that can be identified with techniques such as 13-C NMR spectroscopy. The direct measurement of chemical structural features, along with the use of the measured features rather than correlations, seems to be the best approach to identify specific behaviors of coals for industry.

Since the model accurately treats the chemical features of the coal, nitrogen release models developed from this foundation will have a more fundamental basis and hence have the potential to be more generally applicable than empirically-based models. The nitrogen released during devolatilization has a major impact on NOx reduction strategies, and is very coal dependent. Therefore, as more is learned about the chemical forms of nitrogen in coal, tar, and char, the CPD model provides an ideal tool for testing new theories regarding nitrogen release. Since the CPD model is already incorporated into comprehensive boiler simulation codes, the new information from this contract can be easily used by industry.

In addition to coal pyrolysis, the foundation of chemical structure and reaction mechanisms in the CPD model have potential for application to modeling liquefaction processes. Many of the key features of liquefaction have parallel components in coal pyrolysis, and since the chemical structure is correctly modeled, the transition to liquefaction modeling is seen as promising.

The CPD model was developed in FORTRAN on a VAX system, but has been demonstrated to work on Unix-based HP workstation and Convex computer environments. Typical calculations are performed in less than 1 CPU second on an HP, and the code performs well on a personal computer.

Two versions of the CPD model are available: (1) particle temperature versus particle residence time are required input; and (2) gas temperature and particle residence time are required input. Version (2) solves the particle energy equation and calls the CPD model as a subroutine. This version includes effects of convective heating, simple radiative heat exchange with the walls, and effects of high mass transfer.

The CPD model has been distributed internationally, and has been included into two publicly available comprehensive boiler simulation codes, including:

Richard Buckius	Univ. of Illinois
M. Pourkashanian	Univ. of Leeds, England
Woody Fiveland	Babcock & Wilcox
Michael Groenhout	Airflow Sciences
Lasse Sorensen	RISO National Laboratory, Denmark
Terry Wall	University of Newcastle, Australia

and in PCGC-3 (Pulverized Coal Combustion and Gasification - 3-Dimensional; developed at Brigham Young University) and Fluent (the largest commercial code for 3-D computational fluid dynamics in the world).

The CPD model was developed by Thomas H. Fletcher and Alan R. Kerstein at the Combustion Research Facility, Sandia National Laboratories, Livermore, California 94551-0969, and Ronald J. Pugmire, Mark Solum, and David M. Grant, Departments of Fuels Engineering and Chemistry, University of Utah, Salt Lake City, Utah 84112, with some follow-on work performed by Dr. Fletcher where he is currently with the BYU Department of Chemical Engineering. The research at Sandia was supported by the Department of Energy's Pittsburgh Energy Technology Center's Direct Utilization AR&TD;Program and the DOE Division of Engineering and Geosciences through the Office of Basic Energy Sciences. The research at the University of Utah was supported by the National Science Foundation through the Advanced Combustion Engineering Research Center (ACERC) at Brigham Young University and the University of Utah, by the Department of Energy Division of Chemical Sciences, Office of Basic Energy Sciences, and by the Associated Western Universities (AWU) who provided summer faculty fellowships for Professors Pugmire and Grant to spend time at Sandia. Funds for the ACERC center are also received from the State of Utah, 75 industrial participants, and the U.S. Department of Energy.

1. Grant, D. M., R. J. Pugmire, T. H. Fletcher, and A. R. Kerstein, "A Chemical Model of Coal Devolatilization Using Percolation Lattice Statistics," Energy and Fuels, 3, 175 (1989).

2. Fletcher, T. H., A. R. Kerstein, R. J. Pugmire, and D. M. Grant, "Chemical Percolation Model for Devolatilization: II. Temperature and Heating Rate Effects on Product Yields," Energy and Fuels, 4, 54 (1990).

3. Fletcher, T. H., A. R. Kerstein, R. J. Pugmire, M. S. Solum, and D. M. Grant, " A Chemical Percolation Model for Devolatilization: 3. Chemical Structure as a Function of Coal Type," Energy and Fuels, 6, 414 (1992).

4. Fletcher, T. H., A. R. Kerstein, R. J. Pugmire, M. S. Solum, and D. M. Grant, "A Chemical Percolation Model for Devolatilization: Milestone Report," Sandia report SAND92-8207, available NTIS (May, 1992).

5. Niksa, S. and A. R. Kerstein, "FLASHCHAIN Theory for Coal Devolatilization Kinetics. 1. Formulation," Energy and Fuels, 5, 647 (1991).

6. Solomon, P. R., D. G. Hamblen, R. M. Carangelo, M. A. Serio, and G. V. Deshpande, "General Model of Coal Devolatilization," Energy and Fuels, 2, 405 (1988).


Dr. Fletcher home page , ACERC home page , BYU ChE Dept home page , BYU home page